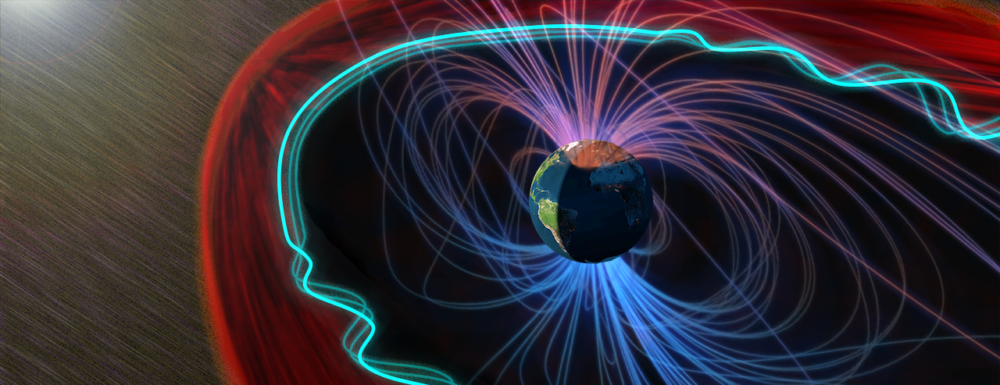
Background
Throughout the heliosphere many different plasma regions with sharp discontinuities at their interfaces are present. For example, Earth’s magnetosphere is bounded by a magnetopause and often also contains a well-defined plasmapause marking the boundary of cold dense plasma outflowing from the ionosphere interface. The dynamics of these boundaries, magnetohydrodynamic (MHD) surface waves (e.g. Kivelson & Chen, 1995), are caused by internally/externally-driven pressure imbalances and/or flow shears. Surface waves are thought to control the flow of mass, energy, and momentum from the solar wind into a magnetosphere – filtering, accumulating, and guiding the turbulent disturbances omnipresent in space plasmas. This transfer will be most efficient at the natural eigenfrequencies of the system. It is the coupling between these discrete modes and the Alfvén and slow magnetosonic continua which makes surface waves in plasmas very different from their counterparts in hydrodynamics and acoustics. Potential impacts may be had on auroral, ionospheric, outer radiation belt, and trapped magnetospheric plasmas – caused either directly (e.g. magnetopause shadowing, Staples+, 2020) or through associated ultra-low frequency (ULF) waves (e.g. diffusion or precipitation, Keiling+, 2016; Rae+, 2018). Surface waves therefore constitute a fundamental component to, and way of probing, the solar-wind–magnetosphere–ionosphere dynamical (SW-M-I) coupling.
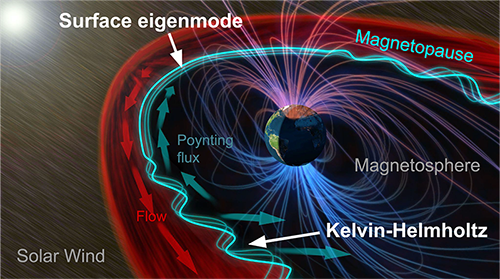
Surface waves at Earth’s magnetosphere have mostly been studied at the magnetopause flanks. Here the fast flows in the magnetosheath may generate/amplify waves via the Kelvin Helmholtz Instability (KHI; Fairfield+, 2000; Otto & Fairfield, 2000) as depicted in Figure 1. The flow largely controls wave frequency in this regime and advects the disturbances tailward. The non-linear evolution of KHI-driven surface waves into vortices leads to important cross-scale (fluid, ion, electron) mass and energy transport mechanisms at both low- and high-latitudes, e.g. heating, reconnection, and kinetic waves (Nykyri+, 2020, 2021).
In contrast to this regime, where flows are negligible the finite extent of magnetospheric field lines (due to anchoring by the highly conductive ionosphere) becomes significant. Poleward propagating surface waves result in reflection, establishing a surface eigenmode (Chen & Hasegawa, 1974; Plaschke & Glassmeier, 2011), as also illustrated in Figure 1 across the dayside magnetopause. Attention has recently returned to this lowest-frequency, largest-scale magnetospheric normal mode. Cutting-edge high-resolution global MHD simulations can now reproduce this natural response to solar wind impulses (Hartinger+, 2015; Archer+, 2021, 2022). These simulations led to high-profile observational discoveries of surface eigenmodes at both Earth’s magnetopause and plasmapause via case studies (Archer+, 2019; He+, 2020).
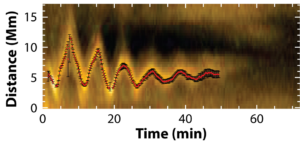
Surface waves are not unique to Earth’s magnetosphere. Observations and modelling suggest they are prevalent at the other planetary magnetospheres too, thus spanning a wide range of scales and plasma conditions across these different environments (e.g. Masters+, 2009; Liljeblad+, 2015; Zhang+, 2018). For example, at Jupiter and Saturn the rapid planetary rotation and corotating magnetodisc result in a very different dynamic interaction and thus unanticipated surface wave results (Ma+, 2015; Zhang+, 2018). Beyond magnetospheres, structures present within the Sun’s atmosphere such as coronal loops, helmet streamers, and plumes are also observed to guide wave perturbations (Nakariakov+, 2016). The sausage and kink modes excited by loop displacements, e.g. from coronal eruptions, share many conceptual similarities to surface eigenmodes. These standing transverse oscillations (see Figure 2) are some of the most intensively studied wave phenomena in the solar corona (Li+, 2013; Guo+, 2019; Yu & Nakariakov, 2020). Surface wave processes are also important in interplanetary space, e.g. affecting the trajectories of Coronal Mass Ejections (Foullon+, 2011, 2013; Nykyri & Foullon, 2013). There is, therefore, great potential for knowledge exchange between the magnetospheric, heliospheric and coronal research communities.
Problem
Numerous fundamental conceptual challenges concerning surface waves remain. Commonly used box models for all MHD waves (Figure 3) are oversimplifications of the magnetospheric environment neglecting:
- The complex geometry of field lines may alter surface waves’ frequencies, structure and/or reflection points (Van Doorsselaere, 2004; Pilipenko+, 2005; Archer+, 2021, 2022).
- Inhomogeneities on both sides of the boundary should non-resonantly couple surface waves to the Alfvén mode leading the wave to have mixed properties (Kozyreva+, 2019; Archer+, 2021, 2022).
- Finite boundary thickness is thought to result in irreversible conversion of surface wave energy, yet to be demonstrated (e.g, Kozyreva+, 2019; Guo+, 2020; Archer+, 2022).
- Turbulent conditions, despite serving as a driving source for surface waves, may damp the collective modes as the system’s properties adjust in response (Pilipenko+, 2017, 2018).
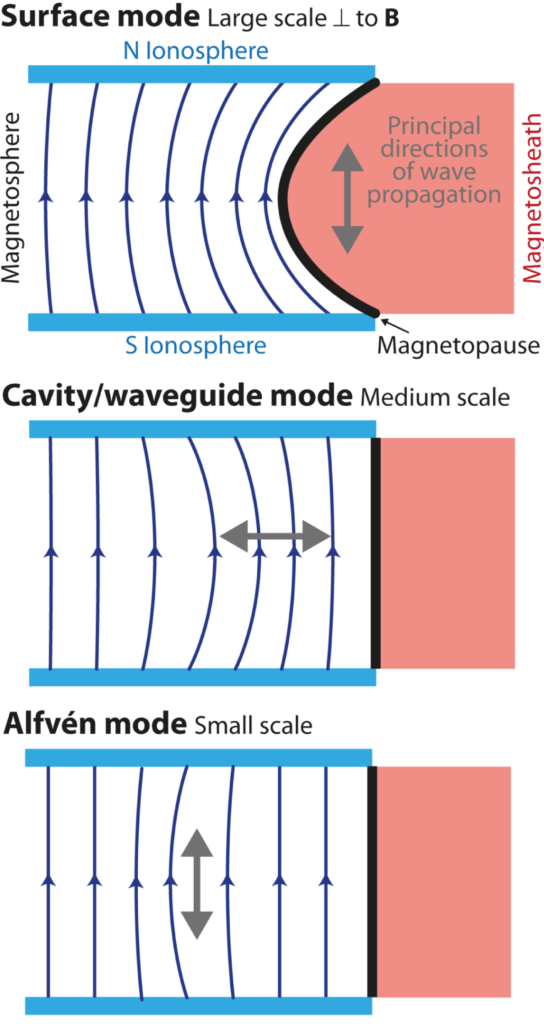
All these factors call into question the reliability of our current theoretical predictions. Further, it is known that the solar wind, magnetosphere, and ionosphere are highly variable environments whose changing properties will affect the frequency, growth/damping rate, and spatial structure of not only surface waves (Archer & Plaschke, 2015; Nykyri, 2012, 2017; Henry, 2017; Leonovich & Kozlov, 2018, 2019) but the other MHD body waves illustrated in Figure 3 also (Archer+, 2015, 2017). Hence, how surface waves couple to body eigenmodes globally, such as Alfvénic field line resonances, and distribute solar wind energy around the magnetosphere is not well established.
Progress has also been hindered due to difficulties in observational detection. Transient responses to external impacts have complicated patterns, due to several processes that drive wave activity (pressure variations, flow shears, reconnection etc.) occurring simultaneously, which are hard to disentangle. These processes can even modify the growth and signatures of one another (Nykyri+, 2003; Ma+, 2014; Walsh+, 2015; Fuselier+, 2019; Hwang+, 2020). Also, internal processes (e.g. the drift mirror instability) can generate compressional ULF waves within the low- and high-latitude magnetospheric boundary layer (e.g., Constantinescu+,2009; Nykyri+ 2021b), which may lead to surface wave growth at the magnetopause, further adding to the difficulty of identifying the root cause. Even unambiguously determining from observations that a surface wave is subject to KHI remains challenging (Keiling+, 2016) with simplified automatic techniques only developed recently (Rice+, 2022). Such observational limitations have led to restrictive criteria for identifying surface waves. For example, the first direct evidence of the magnetopause surface eigenmode required five spacecraft in a string-of-pearls measuring the driver, boundary response, and corresponding ULF waves (Figure 4; Archer+, 2019). This contrasts with MHD body modes, where detection and characterisation is less difficult. Indirect approaches to detecting surface waves under different (and simultaneous) generating processes are thus required. Unfortunately, attempts using spacecraft, ground magnetometer, or auroral observations have collectively proven inconclusive (Plaschke+, 2009; Archer+, 2013; Pilipenko+, 2017, 2018; Kozyreva+, 2019; He+, 2020; Horvath & Lovell, 2021). This is arguably because the expected signatures of surface waves under realistic conditions are not well established. Only with new observational methods based on improved predictions can the numerous outstanding questions about surface waves, such as their efficiency in mediating energy pathways through Earth’s magnetosphere, be determined.